Second Derivative Test – Explanation, Usefulness, Examples, Application, FAQs

Mathematics is the most tricky language of the universe. But what’s more tricky in mathematics is Calculus. One of the most frightening things to many students and mathematics excerpts also is the Second Derivative Test.

Are you also looking for a perfect explanation or a helpful guide of 2nd Derivatives testing? Then you are in the right place today. Keep reading till the end of this guide to know every detail about this convoluted topic from advanced Calculus. 

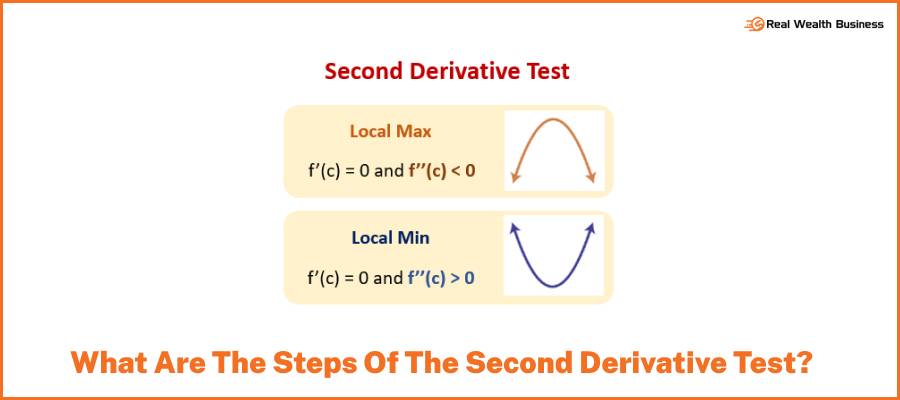
What Is The Second Derivative Test?

Let’s hit the bull’s eye at first before jumping into anything else – What is The Second Derivative Test?

It’s a systematic method related to Calculus that finds the absolute minimum and absolute maximum value of a real-valued function. The second derivative test is defined on the basis of a bounded or closed interval and used in mitigating engineering economics and physics optimization problems.

Keep an eye out on our next sections to learn more about second derivatives testing, including its formula, steps, examples, and several other insights.

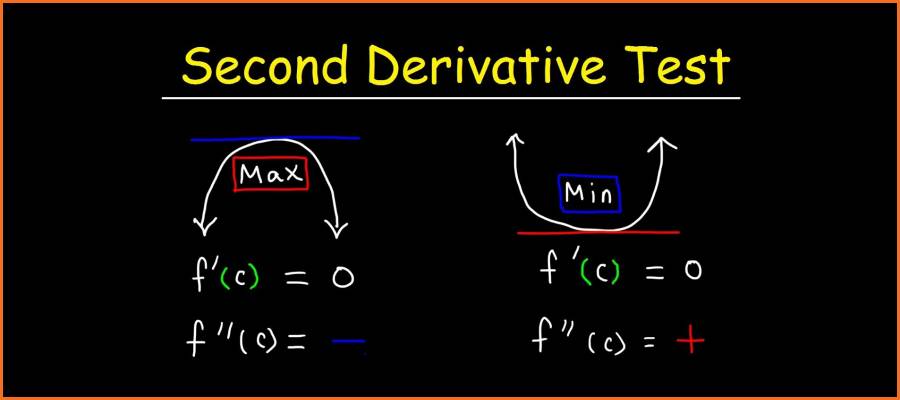
What Are The Steps Of The Second Derivative Test?

I am sure you are pondering on how to find the second derivative test. Well, it may seem a little complicated to you if you are not so brilliant in math. That’s why we have brought a step-by-step guide for you. Follow the points below to determine the local minima and local maxima of the real-valued function:

• Find the first derivative f’(x) of the function f(x). Now equalize it to 0 f’(x) = 0. After that determine two limiting points – x1, x2.
• The second step is to find the second derivative of f’’(x). Then substitute the limiting points. 
• If you get the value of the second derivative testing greater than zero, that is f’’(X1) > 0, X1, which is the limiting point, will be the local maxima. Furthermore, if the second derivative test result is less than zero that is f’’(X1) < 0, the limiting point X2 will be the local maxima.

The above stepwise method is the answer to your question – how to find the second derivative test?

What Benefits You Can Enjoy From The Second Derivative Test?

Here comes the usefulness of Second Derivatives Testing. Does it really make sense if you are solving a problem by applying a specific method without knowing what benefits that method can deliver? So, take a look at the numbered points below without any more ado:

1. The second derivative test formula is used to find both the local minima as well as the local maxima of a function, given the constraints.
2. The second derivatives testing helps in finding the minimum or maximum value of a function. Therefore, you become able to find the best solution for the given mathematical problem.
3. When it comes to a parabolic equation, you must know two things very well. The first one is how to find the second derivative along with a strong grip on the second derivative test formula. It will let you know the parabola’s vertex or, in other words, the turning point.
4. In order to detect the extreme points of the curves. The second derivative test formula is immensely helpful.
5. Wanna know whether a curve is concave up or concave down? Close your eyes and go for the second derivative test.

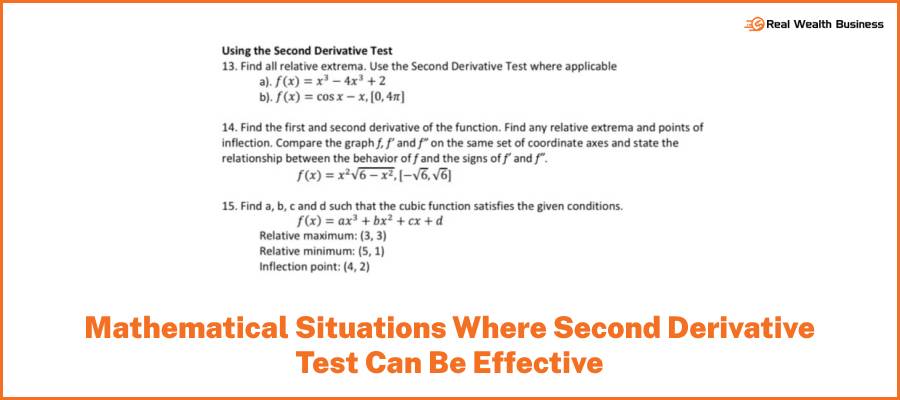
Mathematical Situations Where Second Derivative Test Can Be Effective

Are you confused pondering where exactly you can apply the 2nd Derivative Test? Worry not; we have brought some mathematical problems. These are the perfect scenarios to apply the second derivative test calculator to solve the problems.

• Problem 1: A helicopter of the enemy is traveling along the path defined by the equation P(x) = x + 7, and a soldier placed at the point (1, 2) wants to hit the helicopter. Here to find the minimum distance at which the soldier can hit the helicopter, we can use the second derivative test.
• Problem 2: The profit from a grove of orange trees is given by the expression P(x) = ax + bx2+ cx3 + d, where a, b are constants and x is the number of mango trees per acre. To find the number of mango trees per acre required to maximize the profit we use this second derivative test.
• Problem 3: A ball thrown in the air from the top of a building of height 10m, travels along the path given by the formula h(x) = 60 + x – x2/60., where x is the horizontal distance and h(x) is the height of the ball. To find the maximum height the ball would reach, we use the second derivative test.

Examples Of Second Derivative Test

Now that you have got a sound idea of what the Second Derivative Test is, it’s time to get better clarity. We have put forward two examples of the second derivative test for your better understanding.  

Q1. Find Any Local Extrema Of F(X) = Sin X + Cos X On [0,2π] With The Help Of Second Derivative Test. 

• f′(x) is 0 at x is −2, 0, and 2. 
• Because f″(x) is equal to 12 x 2 −16, you find that f″(−2) = 32 > 0, and f has a local minimum at (−2,−16); 
• f″(2) = 32 > 0, and f has a local maximum at (0,0); and f″(2) is equal to 32 > 0, and f has a local minimum (2,−16).

Q2. Find Any Local Extrema Of F(X) = X 4 − 8 X 2 Using The Second Derivative Test.

• f′(x) = 0 at x = π/4 and 5π/4. 
• Because f″(x) is equal to −sin x −cos x, you find that and f has a local maximum at. 
• Also, and f has a local minimum at

Frequently Asked Questions (FAQ)

Q1. What Does A Derivative Test Tell You?

Derivative test in calculus uses the derivatives of a function in order to locate the critical points of that function. In addition, they also determine if every point is a saddle point, a local maximum, or a local minimum. Apart from all these, you may also get information about the concavity of a function from the derivative tests. 

Q2. What Does The Second Derivative Calculator Test Tell You?

The 2nd derivative test gives us a lot of information about a function. The positive second derivative you get at X tells us that f(x)’s derivative is rising at that specific point. Furthermore, the graph’s curve is concave to that point. As a result, if X  is f(x)’s critical point and the second derivative of the same is positive, then f(x)’s local minimum is X.

Q3. What Does The Third Derivative Tell You?

The third derivative test or high-order derivative test gives you a full classification of the stationary points of a particular function. Its main use is to better the accuracy of the functional approximation.  

Q4. What Is The First Derivative Used For?

From the first derivative of a function, you will get the slope of a tangent line at any instant to the curve. It’s quite obvious that the first derivative gives us more details about a function than that of the 2nd derivative test and 3rd as well.

Q5. Is The Second Derivative Test Always True?

The second derivative test does not always give true results. Especially at the point of inflection, the 2nd derivative test is not true. If that happens, you can go back to the first derivative test as well as cross-check the given values.     

Summing It Up

That’s all about the second derivative tests. To conclude, the usefulness of the second derivative test lies in finding the local minima and local maxima of a function. It applies under a number of constraints that we need to consider. Nevertheless, there are high possibilities that you won’t always get the true results. That is the time when you have to shift to the first derivative test in order to get an accurate result.

Was this article helpful to strengthen your idea about the second derivative test? Do let us know in the comment section if you have got any queries.

Read Also:

• Updated IDRLabs Pooh Pathology Test 2022 – Is It A Mental Disorder Examination?
• What Is A Difficult Person Test? The Best Personality Quiz In 2022
• KTESTONE Colour Personality Test Of TikTok – A Detailed Review
• Updated Borderline Spectrum Test IDRLabs 2022 – An Assessment for BPD!!